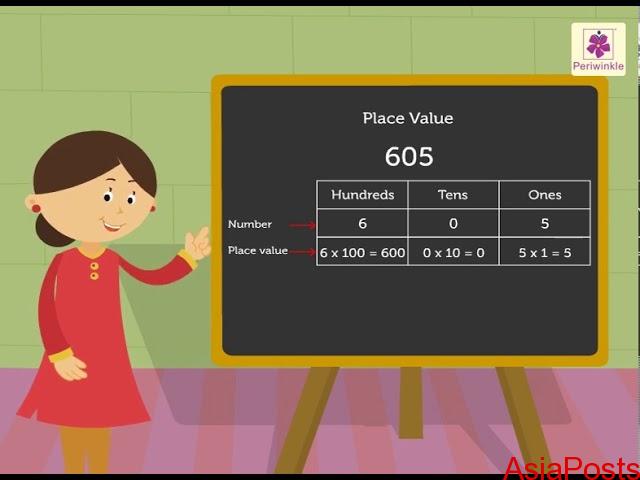
A specific number determines the place value and the values of any numbers. It can only understand a number in mathematics if the number can be deduced numerically.
The value of numbers could be described as a mix of face value and place value. The value of the place is based on its position and is increased from left to right, and it is the value that is the face value of a number.
It is a globally known constant, and any variation in its value is likely. Therefore, let us Distinguish between Place Value and Face Value and assist us in determining the value of a particular number.
Face Value
Face value for a number is the amount of magnitude that a number has naturally, regardless of its position in a numerical form. For instance, in the following number 3526. The face value of digit 2 is 2. It is the standard value of digit 2. This means that it is constant regardless of the circumstances, and any change cannot be considered an integral value.
Place Value
The position of the digit determines the value of the place numeral in an amount. The size of the place value grows as we move from left to left. That means the number on the left-most end of any number is the most value for that particular number.
The value of each place in a number is listed in ascending order, such in the form of one, 100, or 10 thousand or more. Therefore, to determine the value of the place of any number, we need to multiply the amount of face worth the number by what it is worth in its location.
This is demonstrated below. To determine the value of the digit 6 in the 4683 number, we first look at its face value for the number 6. Then, we will multiply it by the value of the location where the digit appears. For instance, the value of the position occupied by the number 6. is 100.
Thus, through multiplication by 100, we can get the value of place 6 in the 4683 number as 600—the value of any number changes as it moves in its zone.
What is the difference between Face and Place Value? Value of a Digit?
There are a lot of numbers we make use of every day. Specific numbers have only one digit, while some have more than one number. They could have ten or more than ten figures, but we use numerals that range from 0 to 9.
Every numeral we use is assigned a face value as well as place and place value. As a result, understanding the difference between a digit’s place value and face value is simple.
The difference between Face and Place Value Value
We must understand the main distinctions between place value as well as face value.
This list shows differences between the face value and place value of a digit within the number.
Let’s have a look.
| Place Value | Face Value |
| 1. Place value is the location of a number in an unspecified number. | The face value of a number in the number is the digit itself. |
| 2. Place value is based on the position of a digit within the number. | The face value does not rely on the position of a number. |
| 3. Place value is the product of the number and position of a digit within the range of a number. | The face value of a number will always remain the same no matter where in the number |
| 4. Value of place for a number equals face value number value of its position | Face value of a digit = the digit in itself |
| 5. The value of zero in any given number is always zero. | Face value for 0 also equals 0. |
| 6. Example: The value of the place 6 in 7632 would be: 6 100 X 600 = 600 | Example Face number 6 of 7632 6 |
How can you tell the difference between value and value?
The difference between value and value is
- The location of a number’s digit is in units, hundreds, tens, thousands, etc.
- Value is the actual value of a number in the number.
- Example: In the number 532, position five is called hundreds, and its value equals 500. In 532, the weight and place were 5 and 500.
The difference between face value using examples
Face value for a number is the digit in itself. However, it can be in any location. It is the difference between the value of a place as well as face value, with examples.
- Each digit is worth ten times the value instantly to their right.
Be aware of this:
- The face value is the same, even though it’s employed in various locations.
- The value of the place changes according to the position of the number.
Example 1
Using the ten basic numbers from 0 and 9, we can display the numbers we wish to.
In the 325th position 2, 2 is on the top in tenth place.
Also, in the number 325 is
- The face value of 2 is 2.
- The value of 2 in the area is 20
Example 2
In the number 235
- As 3 is the 10th position, the value for the place is 3 10 x 3 = 30.
Example 3
In the number 22434
- The face value of all four are the same, which is the face value of all four are the same; that is, the value of the place of 4 at the point of its position is 4. Therefore, the 100’s value is 400.
Example 4
We will now determine if the significance in 9 represents the number 49378.
The place value is calculated by multiplying the face value by the place of a number.
- The face value of 9 is 9.
- In the 49378 number, 9 is located at the location of thousands.
- The face value is 9, and since 9 is at the place of a thousand, this will be 1000. So we receive 9×1000 = 9000
We find the place value 9 = 9000
Conclusion
We are also available to assist you in resolving any concerns. Therefore, please express your questions in the comment section below, and we will react as quickly as possible with answers and a comprehensive step-by-step approach for addressing the problem.